Sr Examen
Otras calculadoras
- Serie de Taylor paso a paso
- Serie de Fourier paso a paso
- Ecuaciones diferenciales paso a paso
- Producto de la serie
-
¿Cómo usar?
- Suma de la serie:
-
6/(n^2-10n+24)
- x^2/(1+n^3*x^3)
-
7/(n^2+n)
-
n/n+1
-
Expresiones idénticas
- (- uno)^(n)/(dos *n- uno)*((x- dos)/ tres)^(dos *n+ uno)
- ( menos 1) en el grado (n) dividir por (2 multiplicar por n menos 1) multiplicar por ((x menos 2) dividir por 3) en el grado (2 multiplicar por n más 1)
- ( menos uno) en el grado (n) dividir por (dos multiplicar por n menos uno) multiplicar por ((x menos dos) dividir por tres) en el grado (dos multiplicar por n más uno)
- (-1)(n)/(2*n-1)*((x-2)/3)(2*n+1)
- -1n/2*n-1*x-2/32*n+1
- (-1)^(n)/(2n-1)((x-2)/3)^(2n+1)
- (-1)(n)/(2n-1)((x-2)/3)(2n+1)
- -1n/2n-1x-2/32n+1
- -1^n/2n-1x-2/3^2n+1
- (-1)^(n) dividir por (2*n-1)*((x-2) dividir por 3)^(2*n+1)
-
Expresiones semejantes
- (-1)^(n)/(2*n+1)*((x-2)/3)^(2*n+1)
- (-1)^(n)/(2*n-1)*((x+2)/3)^(2*n+1)
- (-1)^(n)/(2*n-1)*((x-2)/3)^(2*n-1)
- (1)^(n)/(2*n-1)*((x-2)/3)^(2*n+1)
Suma de la serie (-1)^(n)/(2*n-1)*((x-2)/3)^(2*n+1)
Solución
Ha introducido
[src]
oo ____ \ ` \ n 2*n + 1 \ (-1) /x - 2\ / -------*|-----| / 2*n - 1 \ 3 / /___, n = 1
$$\sum_{n=1}^{\infty} \frac{\left(-1\right)^{n}}{2 n - 1} \left(\frac{x - 2}{3}\right)^{2 n + 1}$$
Sum(((-1)^n/(2*n - 1))*((x - 2)/3)^(2*n + 1), (n, 1, oo))
Radio de convergencia de la serie de potencias
Se da una serie:
$$\frac{\left(-1\right)^{n}}{2 n - 1} \left(\frac{x - 2}{3}\right)^{2 n + 1}$$
Es la serie del tipo
$$a_{n} \left(c x - x_{0}\right)^{d n}$$
- serie de potencias.
El radio de convergencia de la serie de potencias puede calcularse por la fórmula:
$$R^{d} = \frac{x_{0} + \lim_{n \to \infty} \left|{\frac{a_{n}}{a_{n + 1}}}\right|}{c}$$
En nuestro caso
$$a_{n} = \frac{\left(\frac{x}{3} - \frac{2}{3}\right)^{2 n + 1}}{2 n - 1}$$
y
$$x_{0} = 1$$
,
$$d = 1$$
,
$$c = 0$$
entonces
$$R = \tilde{\infty} \left(1 + \lim_{n \to \infty}\left(\left(2 n + 1\right) \left|{\frac{\left(\frac{x}{3} - \frac{2}{3}\right)^{- 2 n - 3} \left(\frac{x}{3} - \frac{2}{3}\right)^{2 n + 1}}{2 n - 1}}\right|\right)\right)$$
Tomamos como el límite
hallamos
$$R^{1} = \tilde{\infty} \left(1 + \frac{9}{x^{2} \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)} - 4 x \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)} + 4 \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)}}\right)$$
$$R^{1} = \tilde{\infty} \left(1 + \frac{9}{x^{2} \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)} - 4 x \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)} + 4 \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)}}\right)$$
$$R = \tilde{\infty} \left(1 + \frac{9}{x^{2} \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)} - 4 x \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)} + 4 \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)}}\right)$$
$$\frac{\left(-1\right)^{n}}{2 n - 1} \left(\frac{x - 2}{3}\right)^{2 n + 1}$$
Es la serie del tipo
$$a_{n} \left(c x - x_{0}\right)^{d n}$$
- serie de potencias.
El radio de convergencia de la serie de potencias puede calcularse por la fórmula:
$$R^{d} = \frac{x_{0} + \lim_{n \to \infty} \left|{\frac{a_{n}}{a_{n + 1}}}\right|}{c}$$
En nuestro caso
$$a_{n} = \frac{\left(\frac{x}{3} - \frac{2}{3}\right)^{2 n + 1}}{2 n - 1}$$
y
$$x_{0} = 1$$
,
$$d = 1$$
,
$$c = 0$$
entonces
$$R = \tilde{\infty} \left(1 + \lim_{n \to \infty}\left(\left(2 n + 1\right) \left|{\frac{\left(\frac{x}{3} - \frac{2}{3}\right)^{- 2 n - 3} \left(\frac{x}{3} - \frac{2}{3}\right)^{2 n + 1}}{2 n - 1}}\right|\right)\right)$$
Tomamos como el límite
hallamos
$$R^{1} = \tilde{\infty} \left(1 + \frac{9}{x^{2} \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)} - 4 x \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)} + 4 \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)}}\right)$$
$$R^{1} = \tilde{\infty} \left(1 + \frac{9}{x^{2} \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)} - 4 x \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)} + 4 \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)}}\right)$$
$$R = \tilde{\infty} \left(1 + \frac{9}{x^{2} \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)} - 4 x \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)} + 4 \operatorname{sign}{\left(x^{2} - 4 x + 4 \right)}}\right)$$
Respuesta
[src]
// / ___________\ \ // / ___________\ \
|| | / 2 | | || | / 2 | |
|| 2 |\/ (-2 + x) | | || 2 |\/ (-2 + x) | |
||-(-2 + x) *atan|--------------| | ||-(-2 + x) *atan|--------------| |
|| \ 3 / | || \ 3 / |
||-------------------------------- for And(x >= -1, x <= 5)| ||-------------------------------- for And(x >= -1, x <= 5)|
|| ___________ | || ___________ |
|| / 2 | || / 2 |
|| 3*\/ (-2 + x) | || 3*\/ (-2 + x) |
2*|< | x*|< |
|| oo | || oo |
|| ____ | || ____ |
|| \ ` | || \ ` |
|| \ n -2*n 2*n | || \ n -2*n 2*n |
|| \ (-1) *3 *(-2 + x) | || \ (-1) *3 *(-2 + x) |
|| / ----------------------- otherwise | || / ----------------------- otherwise |
|| / -1 + 2*n | || / -1 + 2*n |
|| /___, | || /___, |
\\ n = 1 / \\ n = 1 /
- --------------------------------------------------------------- + ---------------------------------------------------------------
3 3
$$\frac{x \left(\begin{cases} - \frac{\left(x - 2\right)^{2} \operatorname{atan}{\left(\frac{\sqrt{\left(x - 2\right)^{2}}}{3} \right)}}{3 \sqrt{\left(x - 2\right)^{2}}} & \text{for}\: x \geq -1 \wedge x \leq 5 \\\sum_{n=1}^{\infty} \frac{\left(-1\right)^{n} 3^{- 2 n} \left(x - 2\right)^{2 n}}{2 n - 1} & \text{otherwise} \end{cases}\right)}{3} - \frac{2 \left(\begin{cases} - \frac{\left(x - 2\right)^{2} \operatorname{atan}{\left(\frac{\sqrt{\left(x - 2\right)^{2}}}{3} \right)}}{3 \sqrt{\left(x - 2\right)^{2}}} & \text{for}\: x \geq -1 \wedge x \leq 5 \\\sum_{n=1}^{\infty} \frac{\left(-1\right)^{n} 3^{- 2 n} \left(x - 2\right)^{2 n}}{2 n - 1} & \text{otherwise} \end{cases}\right)}{3}$$
-2*Piecewise((-(-2 + x)^2*atan(sqrt((-2 + x)^2)/3)/(3*sqrt((-2 + x)^2)), (x >= -1)∧(x <= 5)), (Sum((-1)^n*3^(-2*n)*(-2 + x)^(2*n)/(-1 + 2*n), (n, 1, oo)), True))/3 + x*Piecewise((-(-2 + x)^2*atan(sqrt((-2 + x)^2)/3)/(3*sqrt((-2 + x)^2)), (x >= -1)∧(x <= 5)), (Sum((-1)^n*3^(-2*n)*(-2 + x)^(2*n)/(-1 + 2*n), (n, 1, oo)), True))/3
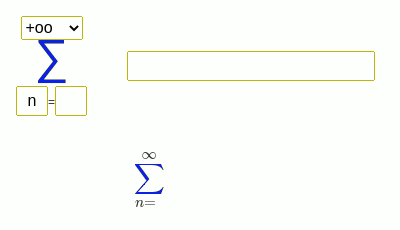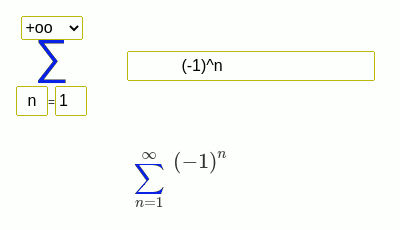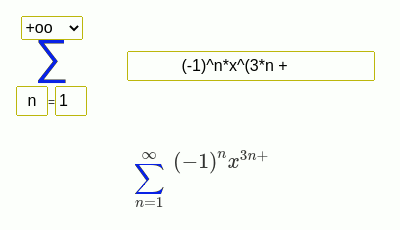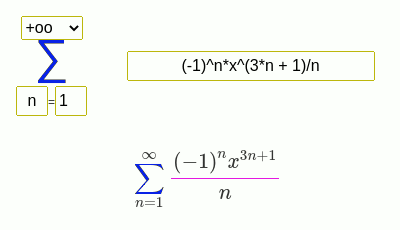
Ejemplos de hallazgo de la suma de la serie
- Suma de la serie de potencias
x^n/n
(x-1)^n
- Factorial
1/2^(n!)
n^2/n!
x^n/n!
k!/(n!*(n+k)!)
- Serie de Flint Hills
csc(n)^2/n^3
- Serie de cuadrados inversos
1/n^2
1/n^4
1/n^6
- Serie armónica
1/n
- Serie de Grandi
(-1)^n
- Serie alternada
(-1)^(n + 1)/n
(n + 2)*(-1)^(n - 1)
(3*n - 1)/(-5)^n
(-1)^(n - 1)*n/(6*n - 5)
- Serie de Newton-Mercator
(-1)^(n + 1)/n*x^n
- Investigar la convergencia de la serie
(3*n - 1)/(-5)^n