Suma de la serie arctg(x)^n(n-1)/(n+1)
Solución
Ha introducido
[src]
oo ____ \ ` \ n \ atan (x)*(n - 1) / ---------------- / n + 1 /___, n = 1
Sum((atan(x)^n*(n - 1))/(n + 1), (n, 1, oo))
Respuesta
[src]
/// 2 2*log(1 - atan(x))\ \ /// 2 2*log(1 - atan(x))\ \ |||- ------- - ------------------|*atan(x) | |||- ------------------ + ------------------|*atan(x) | ||| atan(x) 2 | | ||| 2 2 | | ||\ atan (x) / | ||\ atan (x) - atan(x) atan (x) / | ||---------------------------------------- for And(x >= -tan(1), x < tan(1))| ||--------------------------------------------------- for |atan(x)| < 1| || 2 | || 2 | || | || | || oo | || oo | - |< ____ | + |< ____ | || \ ` | || \ ` | || \ n | || \ n | || \ atan (x) | || \ n*atan (x) | || / -------- otherwise | || / ---------- otherwise | || / 1 + n | || / 1 + n | || /___, | || /___, | || n = 1 | || n = 1 | \\ / \\ /
-Piecewise(((-2/atan(x) - 2*log(1 - atan(x))/atan(x)^2)*atan(x)/2, (x < tan(1))∧(x >= -tan(1))), (Sum(atan(x)^n/(1 + n), (n, 1, oo)), True)) + Piecewise(((-2/(atan(x)^2 - atan(x)) + 2*log(1 - atan(x))/atan(x)^2)*atan(x)/2, Abs(atan(x)) < 1), (Sum(n*atan(x)^n/(1 + n), (n, 1, oo)), True))
Ejemplos de hallazgo de la suma de la serie
- Suma de la serie de potencias
x^n/n
(x-1)^n
- Factorial
1/2^(n!)
n^2/n!
x^n/n!
k!/(n!*(n+k)!)
- Serie de Flint Hills
csc(n)^2/n^3
- Serie de cuadrados inversos
1/n^2
1/n^4
1/n^6
- Serie armónica
1/n
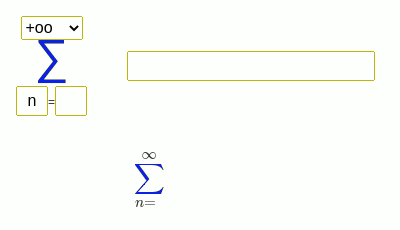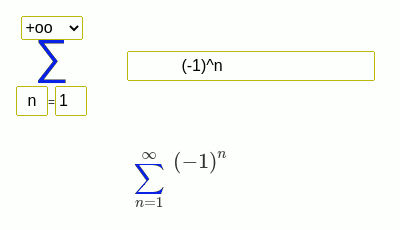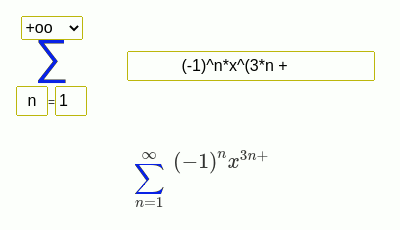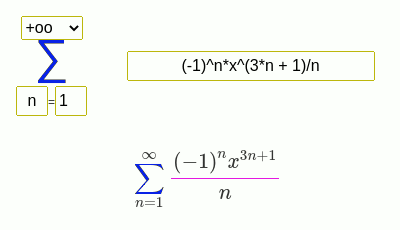
- Serie de Grandi
(-1)^n
- Serie alternada
(-1)^(n + 1)/n
(n + 2)*(-1)^(n - 1)
(3*n - 1)/(-5)^n
(-1)^(n - 1)*n/(6*n - 5)
- Serie de Newton-Mercator
(-1)^(n + 1)/n*x^n
- Investigar la convergencia de la serie
(3*n - 1)/(-5)^n

 n^2/(n+1)
n^2/(n+1)
 n!/2^n
n!/2^n
 n^3/e^n
n^3/e^n