Sr Examen
Otras calculadoras
- Serie de Taylor paso a paso
- Serie de Fourier paso a paso
- Ecuaciones diferenciales paso a paso
- Producto de la serie
-
¿Cómo usar?
- Suma de la serie:
-
n^3/e^n
-
2^n/n^2
-
5
-
(1/2^n)((n+2)/(n(n+2)))
-
Expresiones idénticas
- (n+ uno)^ siete *x^n/n- uno
- (n más 1) en el grado 7 multiplicar por x en el grado n dividir por n menos 1
- (n más uno) en el grado siete multiplicar por x en el grado n dividir por n menos uno
- (n+1)7*xn/n-1
- n+17*xn/n-1
- (n+1)⁷*x^n/n-1
- (n+1)^7x^n/n-1
- (n+1)7xn/n-1
- n+17xn/n-1
- n+1^7x^n/n-1
- (n+1)^7*x^n dividir por n-1
-
Expresiones semejantes
- ((n+1)^7*x^n)/(n-1)
- (n-1)^7*x^n/n-1
- (((n+1)^7)x^n)/(n-1)
- (n+1)^7*x^n/(n-1)
- (n+1)^7*x^n/n+1
- (((n+1)^7)*x^n)/(n-1)
Suma de la serie (n+1)^7*x^n/n-1
Solución
Ha introducido
[src]
oo ____ \ ` \ / 7 n \ \ |(n + 1) *x | / |----------- - 1| / \ n / /___, n = 1
$$\sum_{n=1}^{\infty} \left(-1 + \frac{x^{n} \left(n + 1\right)^{7}}{n}\right)$$
Sum(((n + 1)^7*x^n)/n - 1, (n, 1, oo))
Respuesta
[src]
// / 4 3 2\ \ // x \ // / 3 2\ \ //x*(-1 - x) \ // / 2 \ \ // / 5 2 3 4\ \
// x \ || x*\1 + x + 26*x + 26*x + 66*x / | || -------- for |x| < 1| || x*\-1 - x - 11*x - 11*x / | ||---------- for |x| < 1| || x*\1 + x + 4*x/ | //-log(1 - x) for And(x >= -1, x < 1)\ || x*\-1 - x - 302*x - 302*x - 57*x - 57*x / |
|| ----- for |x| < 1| ||------------------------------------- for |x| < 1| || 2 | ||------------------------------- for |x| < 1| || 3 | ||----------------------- for |x| < 1| || | ||----------------------------------------------- for |x| < 1|
|| 1 - x | || 2 / 4 3 2\ | || (1 - x) | || 2 / 3 2 \ | ||(-1 + x) | || 2 / 2 \ | || oo | || 2 / 5 2 4 3\ |
|| | ||(1 - x) *\1 + x - 4*x - 4*x + 6*x / | || | ||(1 - x) *\-1 + x - 3*x + 3*x/ | || | ||(1 - x) *\1 + x - 2*x/ | || ____ | ||(1 - x) *\-1 + x - 10*x - 5*x + 5*x + 10*x / |
|| oo | || | || oo | || | || oo | || | || \ ` | || |
-oo + 7*|< ___ | + 7*|< oo | + 21*|< ___ | + 21*|< oo | + 35*|< ___ | + 35*|< oo | + |< \ n | + |< oo |
|| \ ` | || ___ | || \ ` | || ___ | || \ ` | || ___ | || \ x | || ___ |
|| \ n | || \ ` | || \ n | || \ ` | || \ 2 n | || \ ` | || / -- otherwise | || \ ` |
|| / x otherwise | || \ 5 n | || / n*x otherwise | || \ 4 n | || / n *x otherwise | || \ 3 n | || / n | || \ 6 n |
|| /__, | || / n *x otherwise | || /__, | || / n *x otherwise | || /__, | || / n *x otherwise | || /___, | || / n *x otherwise |
\\n = 1 / || /__, | ||n = 1 | || /__, | ||n = 1 | || /__, | \\ n = 1 / || /__, |
\\ n = 1 / \\ / \\ n = 1 / \\ / \\ n = 1 / \\ n = 1 /
$$21 \left(\begin{cases} \frac{x}{\left(1 - x\right)^{2}} & \text{for}\: \left|{x}\right| < 1 \\\sum_{n=1}^{\infty} n x^{n} & \text{otherwise} \end{cases}\right) + 7 \left(\begin{cases} \frac{x}{1 - x} & \text{for}\: \left|{x}\right| < 1 \\\sum_{n=1}^{\infty} x^{n} & \text{otherwise} \end{cases}\right) + 35 \left(\begin{cases} \frac{x \left(- x - 1\right)}{\left(x - 1\right)^{3}} & \text{for}\: \left|{x}\right| < 1 \\\sum_{n=1}^{\infty} n^{2} x^{n} & \text{otherwise} \end{cases}\right) + 35 \left(\begin{cases} \frac{x \left(x^{2} + 4 x + 1\right)}{\left(1 - x\right)^{2} \left(x^{2} - 2 x + 1\right)} & \text{for}\: \left|{x}\right| < 1 \\\sum_{n=1}^{\infty} n^{3} x^{n} & \text{otherwise} \end{cases}\right) + 21 \left(\begin{cases} \frac{x \left(- x^{3} - 11 x^{2} - 11 x - 1\right)}{\left(1 - x\right)^{2} \left(x^{3} - 3 x^{2} + 3 x - 1\right)} & \text{for}\: \left|{x}\right| < 1 \\\sum_{n=1}^{\infty} n^{4} x^{n} & \text{otherwise} \end{cases}\right) + 7 \left(\begin{cases} \frac{x \left(x^{4} + 26 x^{3} + 66 x^{2} + 26 x + 1\right)}{\left(1 - x\right)^{2} \left(x^{4} - 4 x^{3} + 6 x^{2} - 4 x + 1\right)} & \text{for}\: \left|{x}\right| < 1 \\\sum_{n=1}^{\infty} n^{5} x^{n} & \text{otherwise} \end{cases}\right) + \begin{cases} \frac{x \left(- x^{5} - 57 x^{4} - 302 x^{3} - 302 x^{2} - 57 x - 1\right)}{\left(1 - x\right)^{2} \left(x^{5} - 5 x^{4} + 10 x^{3} - 10 x^{2} + 5 x - 1\right)} & \text{for}\: \left|{x}\right| < 1 \\\sum_{n=1}^{\infty} n^{6} x^{n} & \text{otherwise} \end{cases} + \begin{cases} - \log{\left(1 - x \right)} & \text{for}\: x \geq -1 \wedge x < 1 \\\sum_{n=1}^{\infty} \frac{x^{n}}{n} & \text{otherwise} \end{cases} - \infty$$
-oo + 7*Piecewise((x/(1 - x), |x| < 1), (Sum(x^n, (n, 1, oo)), True)) + 7*Piecewise((x*(1 + x^4 + 26*x + 26*x^3 + 66*x^2)/((1 - x)^2*(1 + x^4 - 4*x - 4*x^3 + 6*x^2)), |x| < 1), (Sum(n^5*x^n, (n, 1, oo)), True)) + 21*Piecewise((x/(1 - x)^2, |x| < 1), (Sum(n*x^n, (n, 1, oo)), True)) + 21*Piecewise((x*(-1 - x^3 - 11*x - 11*x^2)/((1 - x)^2*(-1 + x^3 - 3*x^2 + 3*x)), |x| < 1), (Sum(n^4*x^n, (n, 1, oo)), True)) + 35*Piecewise((x*(-1 - x)/(-1 + x)^3, |x| < 1), (Sum(n^2*x^n, (n, 1, oo)), True)) + 35*Piecewise((x*(1 + x^2 + 4*x)/((1 - x)^2*(1 + x^2 - 2*x)), |x| < 1), (Sum(n^3*x^n, (n, 1, oo)), True)) + Piecewise((-log(1 - x), (x >= -1)∧(x < 1)), (Sum(x^n/n, (n, 1, oo)), True)) + Piecewise((x*(-1 - x^5 - 302*x^2 - 302*x^3 - 57*x - 57*x^4)/((1 - x)^2*(-1 + x^5 - 10*x^2 - 5*x^4 + 5*x + 10*x^3)), |x| < 1), (Sum(n^6*x^n, (n, 1, oo)), True))
Ejemplos de hallazgo de la suma de la serie
- Suma de la serie de potencias
x^n/n
(x-1)^n
- Factorial
1/2^(n!)
n^2/n!
x^n/n!
k!/(n!*(n+k)!)
- Serie de Flint Hills
csc(n)^2/n^3
- Serie de cuadrados inversos
1/n^2
1/n^4
1/n^6
- Serie armónica
1/n
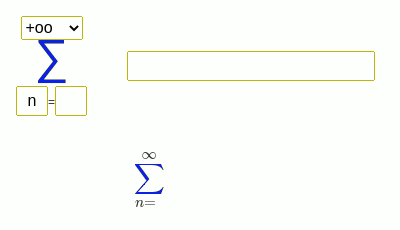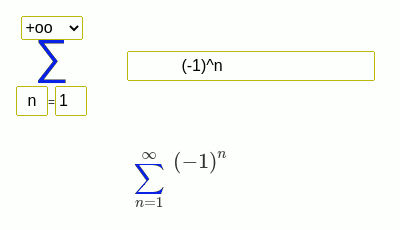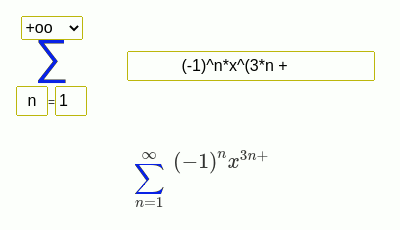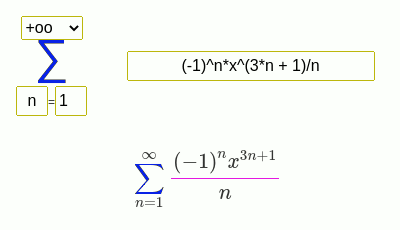
- Serie de Grandi
(-1)^n
- Serie alternada
(-1)^(n + 1)/n
(n + 2)*(-1)^(n - 1)
(3*n - 1)/(-5)^n
(-1)^(n - 1)*n/(6*n - 5)
- Serie de Newton-Mercator
(-1)^(n + 1)/n*x^n
- Investigar la convergencia de la serie
(3*n - 1)/(-5)^n